Brownian Motion

In the 15 years that I spent at my last school, the caretakers rarely, if ever, dusted the top border of the chalkboard. I used their laziness to my advantage, revealing to students that it was evidence that the chalk particles from my constant erasing were small enough to be deflected upwards by the random motion of air particles, even when the air in the room seemed perfectly still to our senses.

Brownian motion was named after the 19^th century botanist Robert Brown. He noticed that pollen suspended in water performed a chaotic dance, a set of random fluctuations, which he did not realize were caused by the movement of water molecules. Initially, perhaps influenced by Goethe, Brown attributed the agitation to the pollen’s vitality, but he realized that the process was physical and not biological when the same kind of motion was observed among dust particles in a water droplet that had been embedded in quartz for millions of years.

A better understanding of Brownian motion came later that century, when the kinetic theory of matter, proposed by J.C. Maxwell, L. Boltzmann. and R.J.E. Clausius, argued that all matter consists of constantly moving atoms or molecules. Their collisions are responsible for the movement of smoke or dust particles in the air, and this same Brownian motion allows a drop of dye to completely dissolve in a cup of water without stirring. The kinetic theory was highly developed, proposing that the temperature is directly proportional to the average kinetic energy of molecules.

E_k= (3/2) kT, where E_k= average kinetic energy of a molecule, k = Boltzmann’s constant and T = absolute temperature measured in Kelvin. The same constant k was also related to the disorder of a molecular system known as entropy, S:

S = k lnW,      

where W = the number of different molecular arrangements that a system can assume. The greater the number of possibilities, the higher the entropy. Boltzmann committed suicide in 1906, a year after Einstein’s quantitative study of Brownian motion. Apparently the former was prone to depression but opposition to the notion of atoms by heavyweights such as Ostwald may have pushed Boltzmann overboard. Ironically, Einstein’s paper, by design, had opened the doors to experimental verification of the kinetic molecular theory.

Assume that a solute is initially concentrated on a flat surface. As it diffuses a certain distance x, its concentration decreases. In the 19^th century, experiments investigated how the diffusion rate was related to concentration.   Fick's second law revealed that the diffusion rate was directly proportional to the acceleration of concentration along one dimension. Fick had derived that , where D = diffusion coefficient. The solution to this differential equation is:

                                    c =. Notice how that for given time interval, t, a larger diffusion coefficient will lead to a lower concentration at a set distance, x. Also as both t and x increase, the concentration is lowered.

If N = total amount of diffusing substance, we can evaluate a by integrating:

N =

Thus by rearranging the result, a =  and

t2

 

t1

 

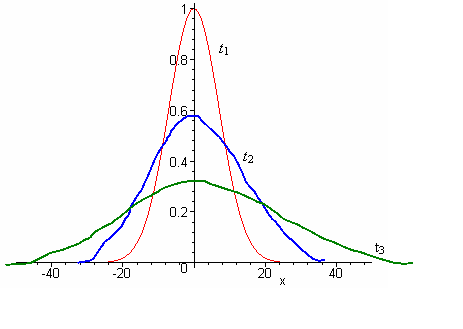
In the above graph of concentration versus displacement at three different time intervals, the area under each curve remains constant as the total amount of solute remains unchanged. Since particles can move either to the right or the left of the membrane, their average displacement would be zero. To avoid this impractical result, we could take the average squared displacement, ² =

Or =--in other words the average displacement of a diffusing particle is directly proportional to the square root of time.

This so-called random walk equation allowed Perrin to experimentally determine a value for the diffusion coefficient, which varies from solute to solute. Painstakingly he and his associates watched, under a microscope, particles experiencing Brownian motion. They obtained enough data of average position (which they squared) versus time, and the slope of the linear relationship was the diffusion coefficient.

Einstein had also argued that a particle being knocked around by its solvent molecules is also being slowed down by the “resistance to flow” or viscosity of the solvent. From the kinetic theory the force of diffusion is given by:

   , (this expression is basically work/ distance = force)

   where G = Gibb’s free energy; R = universal gas constant; and N_A = Avogadro’s number. Note that R/ N_A can also be expressed as Boltzmann’s constant. But working against the diffusion force is the frictional one, F_f, which is a product of a frictional coefficient, f, and the velocity of the particle, v or dx/dt, the particles instantaneous rate of change with respect to time.

            F_f = 6prh(dx/dt), where  f =6prh.

            When the forces are at equilibrium,

            dG/dx = F_f.

            or

            Rearranging,

            But the product of concentration and the rate of change of position is really the rate of diffusion as expressed in Fick’s first law. As a result,

 From the above we conclude that D = .

In Perrin’s time, R was known; he could measure temperature; we‘ve already mentioned how he obtained D, and since he was able to experimentally measure the frictional coefficient, he had a way of estimating Avogadro’s number. Since the value he obtained agreed with other methods that were not dependant on the kinetic molecular theory, it validated the assumptions made by the brilliant but hapless Boltzmann.

Thanks to Einstein, Perrin was able to convince the skeptics that the dance of the molecules was not imaginary.

Printed References

Atkins,P.W. Physical Chemistry. Freeman. 1990

Barrow,Gordon M. Physical Chemistry. McGraw Hill. 1979

Brownian Motion. Scientific American. February 1985

Home